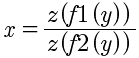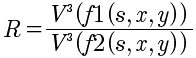Bogus mathematics, (was Re: L'état de PCT, c'est moi (was ...))

MartinT · July 19, 2018, 3:09pm

[Martin Taylor 2018.07.19.10.27]

      [Bruce Nevin
2018-07-19_09:46:11 ET]

Martin Taylor 2018.07.17.17.16 –

Nor am I.

          Rick, Martin listed "eight ... falsehoods you
incorporated in your rebuttal." You replied “they are not
“falsehoods” but the best we could do to understand your
criticisms.” That seems to affirm that you did not
understand his criticisms very well.Â

          I know of two ways to demonstrate understanding,
and both of them involve a test of understanding that is
akin to the Test for controlled variables. One of the two
ways is to apply what is understood. This demonstrates
control of the perceptions intended by the words. The
other way is to paraphrase in different words and ask if
the paraphrase is correct. This is similar to e.g. the
Coin Game.

          Would it be a fair paraphrase of your to enclose
each of the eight pairs (statements in Martin’s list and
your rejoinders to them) in this frame?

          When you said [quote from Martin's rebuttal] it
appeared to us that you meant [quote from your rebuttal of
the rebuttal]. Is that what you intended? If so, [further
rebuttal].

          You actually did this, in effect, at this point

of your reply:

      It seems to me, naively,
that this is not an accurate paraphrase. I think Martin’s
point isÂ

        a. that one form of the
equation is a generalization across all possible
velocities,Â

        b. that the other form of
the equation can be applied only to particular velocity data
from a particular experiment, andÂ

        c. that you employed the
latter (b) as though it were equivalently (a) a
generalization across all possible velocities.

      Only Martin can say whether
or not I have accurately paraphrased what he wrote. If he
affirms that I did, are these paraphrase statements incorrect?

Nearly, but not quite. In your (a) there are not two forms of one

equation, but two different equations that use the standard
Cartesian expression for velocity, namely v=sqrt((dx/dt)²+(dy/dt)² ).
One equation (my 4) is simply a restatement of the fact that when an
experimenter measures dx/dt and dy/dt for something that moves,
these numbers can be used to produce a velocity (and, incidentally,
a direction of movement). That velocity is a particular finding for
a one-time movement.

In your (b) my equation (5) is a standard expression for determining

a radius of curvature. If you take any specific curved track and
move along it at arbitrary velocities and changes of velocity, this
expression will give you the same result for the curvature no matter
how the velocity changed. My equations 1, 2, and 3 – all quite
standard – show why this is the case. My equation (3) shows that
one expression for the radius of curvature at a point is V³ /D,
where D is Marken and Shaffer’s “cross-product correction factor”.
They use D to show that the “correct” value of the power law is R^1/3
= V, and that people report other values of the power only because
they did not know to use this obscure (!) correction factor, D that
they “discovered”.

Your (c) is correct as is.

      I think you have a kind of
important typographical error here:

      I think you meant to say
“we said that your critique was based on your misunderstanding
of those equations.” Is that correct?

I leave it to Rick to answer that one. I interpreted them as meaning

what was written in the first sentence of the quote, that I thought
that they misunderstood the equations. I did, and do, think so.
However, Rick’s second and third sentences in your quote contain the
falsehood. My claim was never that the derivatives were different.
They aren’t. That I said they were is the falsehood. The claim is
actually what you paraphrased above, that the values substituted in
the expression for R need not be the values found in some particular
movement trace. It is merely convenient to use those readily
available values of the derivatives, whereas Marken and Shaffer
proceed as though ONLY those values could legitimately be used in
the expression.

  Are there possibly other misstatements
confusing the discussion?

Yes, many.

Martin

···

Rick Marken
2018-07-17_10:31:31 --â€‹

                    This dispute

seems at last to be converging toward common
perceptions of what is in dispute, but I still
am not understanding it.

                RM: What you are saying is that we made the mistake

of taking the dot derivatives in the two
Gribble/Ostry equations as being time derivatives.

RM: No,
â€‹â€‹

              we said that your critique was based on our

misunderstanding of those equations. Specifically that
the derivatives in the curvature equation were
different from those in the velocity equation. Your
claim that these derivatives are different is simply
wrong and, thus, invalidates your mathematical
critique from the get go.

                [Rick Marken

2018-07-17_10:31:31]

[Martin Taylor 2018.07.16.15.12]

                    MT: As well you have known for a very long time,
I have insufficient hubris to attempt a model of
observed behaviour before trying the TCV to
figure out what variable(s) might be being
controlled during the task. I have no means to
do the TCV needed, so I refrain from suggesting
a model. You are not so inhibited.

                  RM: You have to have had some idea of what the

controlled variable might be when people make
curved movements or you wouldn’t know that the
power law is "almost certainly a side-effect inÂ any
of the experiments that find velocity to have a
near power-law relationship to the radius of
curvature ", as you note in your rebuttal.
In PCT, a “side-effect” is a relationship between
variables that exists because a variable is under
control but this relationship not part of the
process that results in control of that variable.
For example, the relationship between disturbance
and output in a tracking task is a side effect of
controlling the position of the cursor but is not
part of the process that results in control of
cursor position. In order to know that the power
law is, indeed, a side-effect, you had to have an
idea of what variable is under control when people
make curved movements as well as having an idea of
how the instantaneous curvature and velocity of
these movements are related to this variable. This
should have been enough to let you develop a first
approximation to a model of curved movements that
would demonstrate why the instantaneous
curvature and velocity of these movements is a
side effect of controlling this variable. The
model itself would have been a basis for the
TCV; it would be a test of the correctness of
your hypothesis regarding the variable under
control.Â So it would not have been hubris
to model the behavior before doing the TCV since
you presumably had to have had the essential
components of the model in mind when you said that
the power law is almost certainly a side effect.Â

                    MT: For the record, here
are just eight of the falsehoods you
incorporated in your rebuttal of my comment on
the Marken and Shaffer paper (copied from
[Martin Taylor 2018.03.08.23.07]). Despite
having been made aware of their falsity, yet you
continue to repeat some of them on CSGnet. Why
do you do that?

                  RM: BecauseÂ  they are not "falsehoods" but the

best we could do to understand your criticisms.

Â

                    ----------begin quote
(replacing references to “you” with references
to “they”, and added numbering)-------
                    *                          MT: (1) In the very first paragraph you claim
that my reason for writing a critique was that
the idea that the power law might be a
behavioural illusion caused “consternation”,
whereas I made explicit that nothing in my
critique had any bearing on that issue.
Indeed, I finished my critique with the
statement that perhaps the power law is indeed
a behavioural illusion, though M&S sheds
no light on that issue.*

                  RM: Since, as I noted above, you came up with

no hypothesis about what variable might be
controlled, I dismissed your claims of accepting
that the power law is a behavioral illusion
because you gave no evidence of understanding what
a behavioral illusion is.

Â

                     MT: (2) M&S say that
my critique of their use of Gribble and
Ostry’s equations is based on my belief that
those equations are wrong or misleading,
whereas I pointed out that they are well known
and universally accepted equations for using
observed data to measure the velocity
(equation 1) and curvature (equation 2)
profiles observed in an experiment. Neither
Gribble and Ostry nor (so far as I know)
anyone other than Marken and Shaffer ever
claimed that the observed velocity was the
only velocity that could be used to get the
correct curvature from the equation for R.*

                  RM: No, we said that your critique was based on

our misunderstanding of those equations.
Specifically that the derivatives in the curvature
equation were different from those in the velocity
equation. Your claim that these derivatives are
different is simply wrong and, thus, invalidates
your mathematical critique from the get go.

Â

                     MT: (3) I never said
that the derivation of V = R**^1/3D^1/3** was wrong. I said that since the formula for D
was velocity (V) times a constant in spatial
variables, the equation is not an equation
from which one can determine V. The M&S
claim that it is an equation from which one
can determine V is the core of my critique.*

                  RM: And we never said that you said that the

derivation of * V
= R**^1/3D^1/3**Â * was
wrong. We said that what you said about it not
being an equation that can be used to predict V
using linear regression is wrong. Which it is.Â

                     MT: (4) M&S falsely claim that I
argue that “it should have been obvious that
X-dot and Y-dot are derivatives with respect
to time in the expression for V, whereas they
are derivatives with respect to space in the
expression for R (p. 5)”. On the contrary, I
devote the first couple of pages of my
critique to showing why, despite the radius of
curvature being a spatial property,
nevertheless it is quite proper to use time
derivatives in the formula for R.*

RM: But that’s what you argued, right here:Â

                  RM: What you are saying is that we made the

mistake of taking the dot derivatives in the two
Gribble/Ostry equations as being time derivatives.
But that was not mistake. The mistake is all
yours.

                     MT: (5) M&S say that
because Gribble and Ostry correctly
transformed Viviani and Stucchi’s expression
for R using spatial derivatives into one using
time derivatives (a derivation with which I
started my comment), therefore they were
correct to say that ONLY the velocity found in
an experiment can be substituted into the
numerator of the expression for R, whereas
both my derivation and that of Viviani and
Stucchi (essentially the same) makes it
crystal clear that this is not true.*

                  RM: Well, that would be news to all the power

law researchers who computed velocity and
curvature the way I did in my analyses, using time
derivatives.

Â

                     MT: (6) M&S follow
this astounding assertion with an couple of
paragraphs to show why the V = R**^1/3D^1/3** equation is correct, implying that my comment
claimed it to be wrong. Early in my comment,
however, I wrote: “They then write their key
Eq (6) [V = R**^1/3D^1/3** ],
which is true for any value of V whatever…”
Any implication that my comment claimed the
equation to be incorrect is false.*

                  RM: What we showed is that that equation has

been used by others to show what we showed in our
paper – that using only R (curvature) as the
predictor in a regression on V (speed) – will
result in an estimate of the power coefficient of
R that deviates from 1/3 by an amount proportional
to the correlation between R and D (radial
velocity).Â

Â

                     MT: (7) Omitted Variable
Bias: My comment demonstrated that the finding
predicted and reported by M&S was actually
a tautology having no relation to experimental
findings, which will always produce the result
claimed by M&S to be an experimental
result. M&S in the paper and in the
rebuttal treat it as a discovery that can be
made only by careful statistical analysis, and
do not acknowledge the tautology criticism at
all.*

                  RM: Your demonstration that our findings are a

“tautology” made no sense to us. You made this
claim based on your derivation of an equation for
V of the form V = V. But this is true for any
equation. If X = f(Y) then you can substitute X
for the right side of the equation and write the
equation X = X. That’s not a tautology; that’s
just an irrelevant observation.

                     MT: (8) M&S: "At the heart of the
criticisms of our paper by Z/M and Taylor is
the assumption that the power law is a result
of a direct causal connection between
curvature and speed of movement or between
these variables and the physiological
mechanisms that produce them." I have no idea
how this astonishing statement can be derived
from my exposition of the mathematical and
logical flaws in their paper. My comment is
designed to refute exactly M&S’s claim of
my motivation. The comment shows that there is
NO necessary relationship, causal connection
or otherwise, between curvature and speed of
movement.
*

                  RM: You were apparently trying to show,

mathematically, that the curvature and velocity of
a curved movement are physically independent, like
the disturbance and output in a tracking task.
Since you didn’t speculate about the controlled
variable that might be simultaneously affected by
these two variables I assumed that you were dong
this to justify the assumptions of power law
researchers that these two variables are either
causally related or simultaneously caused by a
third variable.Â

Â

--------end quote-------
                    MT: I repeat from my last message: *"*                        What's
the advantage to you of refusing to deal with
scientific points people bring up about your
work?"

                  RM: We dealt with your confusing rebuttal as

best we could. There was nothing scientific about
it inasmuch as it was purely mathematical.

Best

Rick

Â

                    Well, I guess predictions aren't always

wrong, and I am indeed not surprised.

                        Martin

–
Richard S.
MarkenÂ

                                          "Perfection

is achieved not when you
have nothing more to add,
but when you
have
nothing left to take
away.â€?
Â
Â Â Â Â Â Â Â Â Â Â Â Â Â
Â Â --Antoine de
Saint-Exupery

Best

Rick

Â

                                      What's
the advantage to you of
refusing to deal with
scientific points people bring
up about your work? In what
perception you control would
it create error if you were to
accept normal mathematics or
physics as being valid? When
your work is good, it’s good,
but when you make a mistake,
why does it seem so difficult
for you to correct it? In the
curvature paper none of the
criticisms were relevant to a
PCT interpretation, but you
make out that all of them were
intended to refute a “correct
PCT analysis” of the
experimental findings. Why?
                                      I don't expect an answer to a
question raised, but I
wouldn’t be surprised at an
answer to something completely
different.

MartinT · July 19, 2018, 9:15pm

[Martin Taylor 2018.07.19.14.09]

Of course, that is NOT at all what I showed, though it is indeed a

corollary of what I actually showed. Marken and Shaffer already had
used that expression or its equivalent, so even if I had wanted to
demonstrate its truth, there would have been no need. Let’s go
through what I did equation by equation.Â Since we are talking about
my comment and not your rebuttal, I’ll use my numbering.

(1) is the standard expression for R in purely spatial variables,

those being the x,y coordinates of a place along a curve, and s
being the distance along the curve to that point from some arbitrary
zero point. Simple physical argument indicates that a description of
a spatial quantity (such as curvature or radius of curvature) must
be a function of purely spatial variables, and if non-spatial
variables are used, for convenience, they must cancel out of the
expression actually used for the calculation.

(2) shows how this cancellation works for the substitution of an

arbitrary parameter “z” that is a function of “s”. It shows that no
matter what z might be, if it has a continuous derivative dz/ds or
the inverse ds/dz, the expression for R in (1) can be transformed
into the equivalent form in z. Depending on the direction the
equivalence is shown, numerator and denominator each have a
multiplying factor (ds/dz)³ or (dz/ds)³ . These
multipliers cancel out, which is why the substitution of z for s (or
vice-versa) produces the same result for any z.

In (3), z is taken to be the time it takes for an object that moves

arbitrarily along the curve without stopping or retracing to reach
the point at which the derivatives are taken. In this case, the
numerator of the expression simplifies to (ds/dt)³ = V³ .
In this equation and the last equivalence of (2), the denominator is
Marken and Shaffer’s “cross-correlation correction factor” D. If the
argument so far has not made it clear that D is V3*f(x,y,s),
equation (7) later demonstrates it explicitly. As is necessarily
true from basic physical principles, the explicit calculations
demonstrate that the general point mentioned above for an arbitrary
parameter z holds also if the parameter is time or velocity. The
effects of the added variable (in this case V) cancel out.

Marken and Shaffer choose to ignore the generality of the parameter

substitution and the fact that in their specific substitution of the
measured velocities for a single experimental run V³
cancels out from numerator and denominator of the fraction that is
the expression for R. Instead, they leave V³ explicitly
in the numerator, but hide it in their newly discovered
“Cross-correlation correction factor”. They then use the “cccf” as
though it were independent of V in the rest of their paper.

I think this is, to put it mildly, a little different from what Rick

said above that I showed.

Try again, Rick. I keep hoping to be able to learn something from

one of your postings, but I haven’t won this lottery jackpot yet. If
I have made a mathematical error in my other comments on Marken and
Shaffer, I really would like to know. But you please comment on what
I wrote, rather than on something you invented, as you did in this
case.

Martin

···

[Rick Marken 2018-07-19_09:33:53]

[Martin Taylor 2018.07.17.17.16]

            MT: Rick, could you help me correct my "bogus
mathematics" by pointing out by page and paragraph or by
equation number specifically where the mathematics in my
comment on the Marken and Shaffer paper is “bogus”.

          RM: The mathematics are fine. It's the conclusions that

are wrong.Â A particularly egregious example is your
“proof” that our equation relating V to R and D (V =
R^1/3D^1/3, equation 6 in your paper) is a tautology.Â
You do this by showing (correctly, I assume) that D^1/3 is
equivalent to V(1/(R^1/3))

          so thatÂ 
            V

= R^1/3D^1/3 =Â R^1/3
V*(1/(R^1/3))
which, of course,Â reduces to V=V.Â

RM:

But as I’ve said, that’s true of any equation. The fact
thatÂ
V
= R^1/3D^1/3 can be reduced toÂ V = V doesn’t negate
the value of knowing thatÂ
V
= R^1/3D^1/3. This equation analyzes V into its
components just as simple one way analysis of
variance (ANOVA) analyzes the total variance in
scores in an experimentÂ (MS.total) into two
components, the variance in scores across
(MS.between) and within (MS.within) conditions, so
that MS.total = MS.between + MS.within. This is the
basic equation of ANOVA.Â

RM:

Of course, it’s possible to show that MS.total
= MS.between + MS.within isÂ a
“tautology”:Â MS.total = MS.total. We can do this by noting
that MS.within = MS.total - MS.between so thatÂ
MS.total = MS.between + MS.total - MS.between which,
you’ll note, reduces toÂ
MS.total = MS.total.

          RM:Â  But by analyzingÂ MS.total intoÂ MS.between and

MS.within we can learn some interesting things about the
data by computing the two variance components of MS.total
and forming the ratio MS.between/MS.within, a ratio known
as F (for Sir Ronald Fisher, who invented this analysis
method and, as far as I know, never caught flack from
anyone about the basic equation of ANOVA being a
tautology). Knowing the probability of getting different F
ratios in experiments where the independent variable has
no effect (the null hypothesis), it is possible to use the
F ratio observed in an experiment to decide whether one
can reject the null hypothesis with a sufficiently small
probability of being wrong.Â

RM:

Just as it has proved useful to
analyze the total variance in
experiments (
MS.total) Â into
variance component ( MS.between,
MS.within and sometimes
MS.interaction and MS subjects) it
proved useful to us to analyze the
variance in the velocity, V, of a
curved movement into components, R
and D. This analysis produced the
equation V = R^1/3*D^1/3. R and D
are measures of two different
components of the temporal
variation in curved movement just
as MS.between and MS.within are
measures of two different
components of the variation in the
scores observed in an experiment;
R is the variation in curvature
and D is the variation in affine
velocity.Â

RM:

Our equation says that the
variation in V for a curved
movement will be exactly equal to
R^1/3D^1/3. Linearizing this
equation by taking the log of both
sides we get log (V) = 1/3log (R)
+1/3*log (D) . This equation shows
that if one did a linear
regression using the variables
log(R) and log(D) as predictors
and the variable log(V) as the
criterion, the coefficients of the
two predictor variables would be
exactly 1/3 with an intercept of
0. More importantly, this equation
shows that if the variable log (D)
isomitted fromÂ the regression, the
coefficient of log(R) will not
necessarily be found to be exactly
1/3 and the intercept will not
necessarily be found to be exactly
0. This is where Omitted Variable
Bias (OVB) analysis comes in. This
analysis makes if possible to
predict exactly what a regression
analysis will find the coefficient
of log(R) to be if log(D) is
omitted from the regression.

RM:

This finding is important because
the “power law” of movement is
determined by doing a regression
of log (R) on log (V) using the
regression equation log (V) = k +
b*log(R), omitting the variable
log(D). The term “power law”
refers to the fact that the
results of this regression
analysis consistently finds that
the power coefficient b is close
to 1/3. Our analysis shows that
this is a statistical artifact
that results from having left the
variable log(D) out of the
regression analysis. OVB analyiss
shows that the amount by which the
b coefficient is found to deviate
from 1/3 depends on the degree of
covariation between the variable
included in the regression (log
(R)) and the variable omitted from
the regression (log(D)). Since
both log (R) and log (D) are
measured from data (temporal
variations in the x,y position of
the curved movement) the
covariation between these
variables is easily calculated and
the predicted deviation of the
power coefficient, b, from 1/3 can
be exactly predicted.Â

RM:

The covariation between log (R)
and log (D) depends on the nature
of the curved movement trajectory
itself and has nothing to do with
how that movement was generated.
It is in this sense that the
observed power law is a
“behavioral illusion”, the
illusion being that the relatively
consistent observation of an
approximately 1/3 power
relationship between the curvature
(R) and velocity (V) of curved
movements seems to reveal
something important about how
these movements are produced, when
it doesn’t.Â

RM:

So the fact that the equation V =
R^1/3*D^1/3 can be reduced to V =
V does not negate the value of
analyzing V into its components
any more than the fact that the
equationÂ MS.total
= MS.between + MS.within can be
reduced toÂ MS.total
= MS.total negates the value
of analyzing MS.total into its
components.Â

RM:

There are many other incorrect
conclusions in your rebuttal
to our paper, Martin. But I
think this is enough for now
since your “tautology” claim
(based on our alleged
mathematical mistake) seemed
to be central to your
argument.Â

BestÂ

Rick

            You can do this without referring either to your
rebuttal or to the eight falsehoods that I asked you not
to try to justify at this point. My question is not
about them, but specifically about what in my
mathematics you have shown to be bogus. Your previous
response did not address this question.
            Martin

–
Richard S. MarkenÂ

                                  "Perfection

is achieved not when you have
nothing more to add, but when you
have
nothing left to take away.â€?
Â Â Â
Â Â Â Â Â Â Â Â Â Â Â Â Â
–Antoine de Saint-Exupery

MartinT · July 20, 2018, 1:40pm

[Martin Taylor 2018.07.20.09.32]

I think the quote by Ales that Philip found from exactly two years

ago applies in spades. I find it VERY hard to believe that someone
with your level of education and frequently professed expertise can
with a straight face say “* RM: I really tried to find some
relevance of your mathematical analysis to the research we
described in our power law paper. But I’m not sure there is any
relevance because you don’t seem to understand – or want to
understand – what we did*.”

If, by some chance, you seriously can't find the relevance to your

paper of the fact that the velocity completely cancels out of the
expression for the radius of curvature, I don’t see why anyone would
believe anything you say on the subjects about which you so often
pontificate. Just try thinking. It really can help.

Martin

No.

···

[Rick Marken 2018-07-19_16:57:19]

[Martin Taylor 2018.07.19.14.09]

            MT: Of course, that is NOT at all what I
showed…Since we are talking about my comment and not
your rebuttal, I’ll use my numbering.
            MT: (1) is the standard expression for R in purely
spatial variables, those being the x,y coordinates of a
place along a curve, and s being the distance along the
curve to that point from some arbitrary zero point.
Simple physical argument indicates that a description of
a spatial quantity (such as curvature or radius of
curvature) must be a function of purely spatial
variables, and if non-spatial variables are used, for
convenience, they must cancel out of the expression
actually used for the calculation.

          Â RM:Â  Does this mean that the formulas we used to

calculate R and V (and C and A) from the data are
incorrect?

                          MT: Rick, could you
help me correct my “bogus mathematics” by
pointing out by page and paragraph or by
equation number specifically where the
mathematics in my comment on the Marken
and Shaffer paper is “bogus”.

                        RM: The mathematics are fine. It's the

conclusions that are wrong.Â A particularly
egregious example is your “proof” that our
equation relating V to R and D (V =
R^1/3D^1/3, equation 6 in your paper) is a
tautology.Â You do this by showing
(correctly, I assume) that D^1/3 is
equivalent to V(1/(R^1/3))Â

            (2) shows how this cancellation works for the
substitution of an arbitrary parameter “z” that is a
function of “s”. It shows that no matter what z might
be, if it has a continuous derivative dz/ds or the
inverse ds/dz, the expression for R in (1) can be
transformed into the equivalent form in z. Depending on
the direction the equivalence is shown, numerator and
denominator each have a multiplying factor (ds/dz)³
or (dz/ds)³ . These multipliers cancel out,
which is why the substitution of z for s (or vice-versa)
produces the same result for any z.

          RM: What does this have to do with our analysis? That

is, how does it relate to the findings of our regression
analyses?

            MT: In (3), z is taken to be the time it takes for an
object that moves arbitrarily along the curve without
stopping or retracing to reach the point at which the
derivatives are taken. In this case, the numerator of
the expression simplifies to (ds/dt)³ = V³ .
In this equation and the last equivalence of (2), the
denominator is Marken and Shaffer’s “cross-correlation
correction factor” D. If the argument so far has not
made it clear that D is V3*f(x,y,s), equation (7) later
demonstrates it explicitly. As is necessarily true from
basic physical principles, the explicit calculations
demonstrate that the general point mentioned above for
an arbitrary parameter z holds also if the parameter is
time or velocity. The effects of the added variable (in
this case V) cancel out.

          RM: So why did our regression analyses work so well?

What did we do wrong?

Â

            MT: Marken and Shaffer choose to
ignore the generality of the parameter substitution and
the fact that in their specific substitution of the
measured velocities for a single experimental run V³
cancels out from numerator and denominator of the
fraction that is the expression for R. Instead, they
leave V³ explicitly in the numerator, but
hide it in their newly discovered “Cross-correlation
correction factor”. They then use the “cccf” as though
it were independent of V in the rest of their paper.

          RM: We didn't ignore this. We knew nothing about it.

All we knew was what we found in the reports of research
on the power law. And there was nothing in the literature
about the “generality of the parameter substitution” of
which you speak. And what was, indeed, our newly
discovered “cross correlation” variable (D) turns out to
be a well known parameter of curved movement: affine
velocity.Â

Â

            MT: I think this is, to put it
mildly, a little different from what Rick said above
that I showed.

          RM: I really tried to find some relevance of your

mathematical analysis to the research we described in our
power law paper. But I’m not sure there is any relevance
because you don’t seem to understand – or want to
understand – what we did. This is evidenced by what you
say at the beginning of your mathematical critique of our
work:Â “Accordingly, they assert that measured values of
the power law that depart from 1/3 are in error because
they omit consideration of D”. In fact, we never
“asserted” this. What we demonstrated is that
measured values of the power law coefficient will depart
from 1/3 (for the relationship between R and V and 2/3 for
the relationship between C and A) to the extent that the
variable D, which power law researchers always omit from
the regression analysis, covaries with the curvature
variable (R or C) that is included as the predictor
variable in the analysis.Â

            MT: Try again, Rick. I keep hoping to be able to learn
something from one of your postings, but I haven’t won
this lottery jackpot yet.

          RM: Sure, I'll try again. But you might have better

luck if you would explain, as clearly as possible, how
your mathematical analysis relates to our regression
analysis of actual data from curved movements.

Â

            MT: If I have made a mathematical
error in my other comments on Marken and Shaffer, I
really would like to know. But you please comment on
what I wrote, rather than on something you invented, as
you did in this case.

          RM: As I said before, I don't think you have made any

mathematical errors. I just don’t see the relevance of
your mathematical analysis to what we actually did with
our analysis of actual curved movement data. Did we use
the wrong formulas to calculate instantaneous velocity and
curvature? Did we do the regression incorrectly? Did we
use the wrong variables in the regressions?Â

Best

Rick

Â

                Martin

–
Richard S. MarkenÂ

                                  "Perfection

is achieved not when you have
nothing more to add, but when you
have
nothing left to take away.â€?
Â Â Â
Â Â Â Â Â Â Â Â Â Â Â Â Â
–Antoine de Saint-Exupery

so thatÂ V
= R^1/3D^1/3 =Â R^1/3
V*(1/(R^1/3))
which, of course,Â reduces
to V=V.Â

RM:

But as I’ve said, that’s true of any
equation. The fact thatÂ V
= R^1/3D^1/3 can be reduced toÂ V = V
doesn’t negate the value of knowing
thatÂ V
= R^1/3D^1/3. This equation analyzes
V into its components just as simple
one way analysis of variance (ANOVA)
analyzes the total variance in scores
in an experimentÂ (MS.total) into two
components, the variance in scores
across (MS.between) and within
(MS.within) conditions, so that
MS.total = MS.between + MS.within.
This is the basic equation of ANOVA.Â

RM:

Of course, it’s possible to show that
MS.total
= MS.between + MS.within isÂ a
“tautology”:Â MS.total = MS.total. We can do
this by noting that MS.within = MS.total -
MS.between so thatÂ MS.total = MS.between +
MS.total - MS.between which, you’ll note,
reduces toÂ MS.total = MS.total.

                        RM:Â  But by analyzingÂ MS.total

intoÂ MS.between and MS.within we can learn
some interesting things about the data by
computing the two variance components of
MS.total and forming the ratio
MS.between/MS.within, a ratio known as F
(for Sir Ronald Fisher, who invented this
analysis method and, as far as I know, never
caught flack from anyone about the basic
equation of ANOVA being a tautology).
Knowing the probability of getting different
F ratios in experiments where the
independent variable has no effect (the null
hypothesis), it is possible to use the F
ratio observed in an experiment to decide
whether one can reject the null hypothesis
with a sufficiently small probability of
being wrong.Â

RM:

Just as it has proved
useful to analyze the
total variance in
experiments ( MS.total) Â into
variance component ( MS.between,
MS.within and
sometimes
MS.interaction and
MS subjects) it
proved useful to us
to analyze the
variance in the
velocity, V, of a
curved movement into
components, R and D.
This analysis
produced the
equation V =
R^1/3*D^1/3. R and D
are measures of two
different components
of the temporal
variation in curved
movement just as
MS.between and
MS.within are
measures of two
different components
of the variation in
the scores observed
in an experiment; R
is the variation in
curvature and D is
the variation in
affine velocity.Â

RM:

Our equation says
that the variation
in V for a curved
movement will be
exactly equal to
R^1/3D^1/3.
Linearizing this
equation by taking
the log of both
sides we get log (V)
= 1/3log (R)
+1/3*log (D) . This
equation shows that
if one did a linear
regression using the
variables log(R) and
log(D) as predictors
and the variable
log(V) as the
criterion, the
coefficients of the
two predictor
variables would be
exactly 1/3 with an
intercept of 0. More
importantly, this
equation shows that
if the variable log
(D) isomitted
fromÂ the regression,
the coefficient of
log(R) will not
necessarily be found
to be exactly 1/3
and the intercept
will not necessarily
be found to be
exactly 0. This is
where Omitted
Variable Bias (OVB)
analysis comes in.
This analysis makes
if possible to
predict exactly what
a regression
analysis will find
the coefficient of
log(R) to be if
log(D) is omitted
from the regression.

RM:

This finding is
important because
the “power law” of
movement is
determined by doing
a regression of log
(R) on log (V) using
the regression
equation log (V) = k

b*log(R), omitting
the variable log(D).
The term “power law”
refers to the fact
that the results of
this regression
analysis
consistently finds
that the power
coefficient b is
close to 1/3. Our
analysis shows that
this is a
statistical artifact
that results from
having left the
variable log(D) out
of the regression
analysis. OVB
analyiss shows that
the amount by which
the b coefficient is
found to deviate
from 1/3 depends on
the degree of
covariation between
the variable
included in the
regression (log (R))
and the variable
omitted from the
regression (log(D)).
Since both log (R)
and log (D) are
measured from data
(temporal variations
in the x,y position
of the curved
movement) the
covariation between
these variables is
easily calculated
and the predicted
deviation of the
power coefficient,
b, from 1/3 can be
exactly predicted.Â
```
                                              RM:
```

The covariation
between log (R) and
log (D) depends on
the nature of the
curved movement
trajectory itself
and has nothing to
do with how that
movement was
generated. It is in
this sense that the
observed power law
is a “behavioral
illusion”, the
illusion being that
the relatively
consistent
observation of an
approximately 1/3
power relationship
between the
curvature (R) and
velocity (V) of
curved movements
seems to reveal
something important
about how these
movements are
produced, when it
doesn’t.Â

RM:

So the fact that the
equation V =
R^1/3*D^1/3 can be
reduced to V = V
does not negate the
value of analyzing V
into its components
any more than the
fact that the
equationÂ MS.total
= MS.between +
MS.within can be
reduced toÂ MS.total
= MS.total
negates the
value of
analyzing
MS.total into
its components.Â

RM:

There are many
other incorrect
conclusions in
your rebuttal to
our paper,
Martin. But I
think this is
enough for now
since your
“tautology”
claim (based on
our alleged
mathematical
mistake) seemed
to be central to
your argument.Â

BestÂ

Rick

                          You can do this without referring either
to your rebuttal or to the eight
falsehoods that I asked you not to try to
justify at this point. My question is not
about them, but specifically about what in
my mathematics you have shown to be bogus.
Your previous response did not address
this question.
                          Martin

–
Richard S.
MarkenÂ

                                                "Perfection

is achieved not when
you have nothing
more to add, but
when you
have
nothing left to take
away.â€?
Â
Â Â Â Â Â Â Â Â Â Â
Â Â Â Â Â --Antoine
de Saint-Exupery

MartinT · July 20, 2018, 3:25pm

[Martin Taylor 2018.07.20.10.54]

[Martin Taylor 2018.07.20.09.32]

  I think the quote by Ales that Philip found from exactly two years
ago applies in spades. I find it VERY hard to believe that someone
with your level of education and frequently professed expertise
can with a straight face say “* RM: I really tried to find some
relevance of your mathematical analysis to the research we
described in our power law paper. But I’m not sure there is any
relevance because you don’t seem to understand – or want to
understand – what we did*.”
  If, by some chance, you seriously can't find the relevance to your
paper of the fact that the velocity completely cancels out of the
expression for the radius of curvature, I don’t see why anyone
would believe anything you say on the subjects about which you so
often pontificate. Just try thinking. It really can help.

Here's a hint. If you start off by assuming that a fundamental fact

of physics can be dismissed if the violator is a hierarchic control
system, you will not get many real scientists to believe you. Here’s
an analogy that might help. Does the length of a battery determine
its voltage? Does it become so if a perceptual control system uses
the battery for some purpose?

···

[Rick Marken 2018-07-19_16:57:19]

[Martin Taylor 2018.07.19.14.09]

              MT: Of course, that is NOT at all what I
showed…Since we are talking about my comment and not
your rebuttal, I’ll use my numbering.
              MT: (1) is the standard expression for R in purely
spatial variables, those being the x,y coordinates of
a place along a curve, and s being the distance along
the curve to that point from some arbitrary zero
point. Simple physical argument indicates that a
description of a spatial quantity (such as curvature
or radius of curvature) must be a function of purely
spatial variables, and if non-spatial variables are
used, for convenience, they must cancel out of the
expression actually used for the calculation.

            Â RM:Â  Does this mean that the formulas we used to

calculate R and V (and C and A) from the data are
incorrect?

                            MT: Rick, could you
help me correct my “bogus mathematics”
by pointing out by page and paragraph or
by equation number specifically where
the mathematics in my comment on the
Marken and Shaffer paper is “bogus”.

                          RM: The mathematics are fine. It's the

conclusions that are wrong.Â A
particularly egregious example is your
“proof” that our equation relating V to R
and D (V = R^1/3D^1/3, equation 6 in your
paper) is a tautology.Â You do this by
showing (correctly, I assume) that D^1/3
is equivalent to V(1/(R^1/3))Â

              (2) shows how this cancellation works for the
substitution of an arbitrary parameter “z” that is a
function of “s”. It shows that no matter what z might
be, if it has a continuous derivative dz/ds or the
inverse ds/dz, the expression for R in (1) can be
transformed into the equivalent form in z. Depending
on the direction the equivalence is shown, numerator
and denominator each have a multiplying factor (ds/dz)³
or (dz/ds)³ . These multipliers cancel out,
which is why the substitution of z for s (or
vice-versa) produces the same result for any z.

            RM: What does this have to do with our analysis? That

is, how does it relate to the findings of our regression
analyses?

              MT: In (3), z is taken to be the time it takes for an
object that moves arbitrarily along the curve without
stopping or retracing to reach the point at which the
derivatives are taken. In this case, the numerator of
the expression simplifies to (ds/dt)³ = V³ .
In this equation and the last equivalence of (2), the
denominator is Marken and Shaffer’s “cross-correlation
correction factor” D. If the argument so far has not
made it clear that D is V3*f(x,y,s), equation (7)
later demonstrates it explicitly. As is necessarily
true from basic physical principles, the explicit
calculations demonstrate that the general point
mentioned above for an arbitrary parameter z holds
also if the parameter is time or velocity. The effects
of the added variable (in this case V) cancel out.

            RM: So why did our regression analyses work so well?

What did we do wrong?

Â

              MT: Marken and Shaffer choose to
ignore the generality of the parameter substitution
and the fact that in their specific substitution of
the measured velocities for a single experimental run
V³ cancels out from numerator and
denominator of the fraction that is the expression for
R. Instead, they leave V³ explicitly in the
numerator, but hide it in their newly discovered
“Cross-correlation correction factor”. They then use
the “cccf” as though it were independent of V in the
rest of their paper.

            RM: We didn't ignore this. We knew nothing about it.

All we knew was what we found in the reports of research
on the power law. And there was nothing in the
literature about the “generality of the parameter
substitution” of which you speak. And what was, indeed,
our newly discovered “cross correlation” variable (D)
turns out to be a well known parameter of curved
movement: affine velocity.Â

Â

              MT: I think this is, to put it
mildly, a little different from what Rick said above
that I showed.

            RM: I really tried to find some relevance of your

mathematical analysis to the research we described in
our power law paper. But I’m not sure there is any
relevance because you don’t seem to understand – or
want to understand – what we did. This is evidenced by
what you say at the beginning of your mathematical
critique of our work:Â “Accordingly, they assert that
measured values of the power law that depart from 1/3
are in error because they omit consideration of D”. In
fact, we never “asserted” this. What we demonstrated
is that measured values of the power law coefficient
will depart from 1/3 (for the relationship between R and
V and 2/3 for the relationship between C and A) to the
extent that the variable D, which power law researchers
always omit from the regression analysis, covaries with
the curvature variable (R or C) that is included as the
predictor variable in the analysis.Â

              MT: Try again, Rick. I keep hoping to be able to learn
something from one of your postings, but I haven’t won
this lottery jackpot yet.

            RM: Sure, I'll try again. But you might have better

luck if you would explain, as clearly as possible, how
your mathematical analysis relates to our regression
analysis of actual data from curved movements.

Â

              MT: If I have made a mathematical
error in my other comments on Marken and Shaffer, I
really would like to know. But you please comment on
what I wrote, rather than on something you invented,
as you did in this case.

            RM: As I said before, I don't think you have made any

mathematical errors. I just don’t see the relevance of
your mathematical analysis to what we actually did with
our analysis of actual curved movement data. Did we use
the wrong formulas to calculate instantaneous velocity
and curvature? Did we do the regression incorrectly? Did
we use the wrong variables in the regressions?Â

Best

Rick

Â

                  Martin

–
Richard S. MarkenÂ

                                    "Perfection

is achieved not when you have
nothing more to add, but when
you
have
nothing left to take away.â€?
Â Â
Â Â Â Â Â Â Â Â Â Â Â Â Â Â
–Antoine de Saint-Exupery

so thatÂ V
= R^1/3D^1/3 =Â R^1/3
V*(1/(R^1/3))
which, of course,Â reduces
to V=V.Â

RM:

But as I’ve said, that’s true of any
equation. The fact thatÂ V
= R^1/3D^1/3 can be reduced toÂ V = V
doesn’t negate the value of knowing
thatÂ V
= R^1/3D^1/3. This equation
analyzes V into its components just
as simple one way analysis of
variance (ANOVA) analyzes the total
variance in scores in an experimentÂ
(MS.total) into two components, the
variance in scores across
(MS.between) and within (MS.within)
conditions, so that MS.total =
MS.between + MS.within. This is the
basic equation of ANOVA.Â

RM:

Of course, it’s possible to show
that MS.total
= MS.between + MS.within isÂ a
“tautology”:Â MS.total = MS.total. We can
do this by noting that MS.within =
MS.total - MS.between so thatÂ MS.total =
MS.between + MS.total - MS.between which,
you’ll note, reduces toÂ MS.total =
MS.total.

                          RM:Â  But by analyzingÂ MS.total

intoÂ MS.between and MS.within we can learn
some interesting things about the data by
computing the two variance components of
MS.total and forming the ratio
MS.between/MS.within, a ratio known as F
(for Sir Ronald Fisher, who invented this
analysis method and, as far as I know,
never caught flack from anyone about the
basic equation of ANOVA being a
tautology). Knowing the probability of
getting different F ratios in experiments
where the independent variable has no
effect (the null hypothesis), it is
possible to use the F ratio observed in an
experiment to decide whether one can
reject the null hypothesis with a
sufficiently small probability of being
wrong.Â

RM:

Just as it has
proved useful to
analyze the total
variance in
experiments ( MS.total) Â into
variance component ( MS.between,
MS.within and
sometimes
MS.interaction and
MS subjects) it
proved useful to
us to analyze the
variance in the
velocity, V, of a
curved movement
into components, R
and D. This
analysis produced
the equation V =
R^1/3*D^1/3. R and
D are measures of
two different
components of the
temporal variation
in curved movement
just as MS.between
and MS.within are
measures of two
different
components of the
variation in the
scores observed in
an experiment; R
is the variation
in curvature and D
is the variation
in affine
velocity.Â

RM:

Our equation says
that the variation
in V for a curved
movement will be
exactly equal to
R^1/3D^1/3.
Linearizing this
equation by taking
the log of both
sides we get log
(V) = 1/3log (R)
+1/3*log (D) .
This equation
shows that if one
did a linear
regression using
the variables
log(R) and log(D)
as predictors and
the variable
log(V) as the
criterion, the
coefficients of
the two predictor
variables would be
exactly 1/3 with
an intercept of 0.
More importantly,
this equation
shows that if the
variable log (D)
isomitted fromÂ the
regression, the
coefficient of
log(R) will not
necessarily be
found to be
exactly 1/3 and
the intercept will
not necessarily be
found to be
exactly 0. This is
where Omitted
Variable Bias
(OVB) analysis
comes in. This
analysis makes if
possible to
predict exactly
what a regression
analysis will find
the coefficient of
log(R) to be if
log(D) is omitted
from the
regression.

RM:

This finding is
important because
the “power law” of
movement is
determined by
doing a regression
of log (R) on log
(V) using the
regression
equation log (V) =
k + b*log(R),
omitting the
variable log(D).
The term “power
law” refers to the
fact that the
results of this
regression
analysis
consistently finds
that the power
coefficient b is
close to 1/3. Our
analysis shows
that this is a
statistical
artifact that
results from
having left the
variable log(D)
out of the
regression
analysis. OVB
analyiss shows
that the amount by
which the b
coefficient is
found to deviate
from 1/3 depends
on the degree of
covariation
between the
variable included
in the regression
(log (R)) and the
variable omitted
from the
regression
(log(D)). Since
both log (R) and
log (D) are
measured from data
(temporal
variations in the
x,y position of
the curved
movement) the
covariation
between these
variables is
easily calculated
and the predicted
deviation of the
power coefficient,
b, from 1/3 can be
exactly
predicted.Â

RM:

The covariation
between log (R)
and log (D)
depends on the
nature of the
curved movement
trajectory itself
and has nothing to
do with how that
movement was
generated. It is
in this sense that
the observed power
law is a
“behavioral
illusion”, the
illusion being
that the
relatively
consistent
observation of an
approximately 1/3
power relationship
between the
curvature (R) and
velocity (V) of
curved movements
seems to reveal
something
important about
how these
movements are
produced, when it
doesn’t.Â

RM:

So the fact that
the equation V =
R^1/3*D^1/3 can be
reduced to V = V
does not negate
the value of
analyzing V into
its components any
more than the fact
that the equationÂ MS.total
= MS.between +
MS.within can be
reduced toÂ MS.total
= MS.total
negates the
value of
analyzing
MS.total into
its
components.Â

RM:

There are many
other
incorrect
conclusions in
your rebuttal
to our paper,
Martin. But I
think this is
enough for now
since your
“tautology”
claim (based
on our alleged
mathematical
mistake)
seemed to be
central to
your
argument.Â

BestÂ

Rick

                            You can do this without referring either
to your rebuttal or to the eight
falsehoods that I asked you not to try
to justify at this point. My question is
not about them, but specifically about
what in my mathematics you have shown to
be bogus. Your previous response did not
address this question.
                            Martin

–
Richard
S. MarkenÂ

                                                  "Perfection

is achieved not
when you have
nothing more to
add, but when you
have
nothing left to
take away.â€?
Â
Â Â Â Â Â Â Â Â Â
Â Â Â Â Â Â
–Antoine de
Saint-Exupery

MartinT · July 24, 2018, 5:09pm

[Martin Taylor 2018.07.24.13.05]

···

Rick, Do you intend to respond to my
last message of four days ago? Are you going to apologise and
acknowledge that my maths is not “bogus” or are you going to
explain to me exactly how it is bogus?

  We can't move on until you do one or the other. Until then, your

replies to Warren since my last message are completely irrelevant
to the whole curvature-velocity relationship question.

  Martin

[Martin Taylor 2018.07.20.10.54]

…
  Anyway, this is all irrelevant to the topic, which is that you
asserted in a message to Bruce Nevin that the mathematics in my
comment on your original paper was “bogus”, a fact that I am
unable to corroborate. I asked you to back up that claim by
showing me my error(s). So far, all you have said is that you
assume my route through equations 1,2,3, and 7 is correct (4, 5,
and 6 are copied from your paper, and are irrelevant). So now I
must assume that the bogus part of the mathematics lies elsewhere.
Please tell me where, and forget about its relevance. I just want
to know exactly where the “bogus” appears in my mathematics.
  Of course, there is another alternative, which is that you could
apologise for the slur and acknowledge that my mathematics is not
“bogus”, in which case we can talk about relevance if you want.
But I think that simply reading my published comment should
suffice, if the mathematics is indeed not bogus.
  Martin
    Martin
[Martin Taylor 2018.07.19.14.09]

                              MT: Rick, could
you help me correct my “bogus
mathematics” by pointing out by page
and paragraph or by equation number
specifically where the mathematics in
my comment on the Marken and Shaffer
paper is “bogus”.

                            RM: The mathematics are fine. It's
the conclusions that are wrong.Â A
particularly egregious example is your
“proof” that our equation relating V to
R and D (V = R^1/3D^1/3, equation 6 in
your paper) is a tautology.Â You do this
by showing (correctly, I assume) that
D^1/3 is equivalent to V(1/(R^1/3))Â

                MT: Of course, that is NOT at all what I
showed…Since we are talking about my comment and
not your rebuttal, I’ll use my numbering.
                MT: (1) is the standard expression for R in purely
spatial variables, those being the x,y coordinates
of a place along a curve, and s being the distance
along the curve to that point from some arbitrary
zero point. Simple physical argument indicates that
a description of a spatial quantity (such as
curvature or radius of curvature) must be a function
of purely spatial variables, and if non-spatial
variables are used, for convenience, they must
cancel out of the expression actually used for the
calculation.

              Â RM:Â  Does this mean that the formulas we used to
calculate R and V (and C and A) from the data are
incorrect?

    No.
                (2) shows how this cancellation works for the
substitution of an arbitrary parameter “z” that is a
function of “s”. It shows that no matter what z
might be, if it has a continuous derivative dz/ds or
the inverse ds/dz, the expression for R in (1) can
be transformed into the equivalent form in z.
Depending on the direction the equivalence is shown,
numerator and denominator each have a multiplying
factor (ds/dz)³ or (dz/ds)³ .
These multipliers cancel out, which is why the
substitution of z for s (or vice-versa) produces the
same result for any z.

              RM: What does this have to do with our analysis?
That is, how does it relate to the findings of our
regression analyses?

                MT: In (3), z is taken to be the time it takes for
an object that moves arbitrarily along the curve
without stopping or retracing to reach the point at
which the derivatives are taken. In this case, the
numerator of the expression simplifies to (ds/dt)³
= V³ . In this equation and the last
equivalence of (2), the denominator is Marken and
Shaffer’s “cross-correlation correction factor” D.
If the argument so far has not made it clear that D
is V3*f(x,y,s), equation (7) later demonstrates it
explicitly. As is necessarily true from basic
physical principles, the explicit calculations
demonstrate that the general point mentioned above
for an arbitrary parameter z holds also if the
parameter is time or velocity. The effects of the
added variable (in this case V) cancel out.

              RM: So why did our regression analyses work so

well? What did we do wrong?

Â

                MT: Marken and Shaffer choose
to ignore the generality of the parameter
substitution and the fact that in their specific
substitution of the measured velocities for a single
experimental run V³ cancels out from
numerator and denominator of the fraction that is
the expression for R. Instead, they leave V³
explicitly in the numerator, but hide it in their
newly discovered “Cross-correlation correction
factor”. They then use the “cccf” as though it were
independent of V in the rest of their paper.

              RM: We didn't ignore this. We knew nothing about
it. All we knew was what we found in the reports of
research on the power law. And there was nothing in
the literature about the “generality of the parameter
substitution” of which you speak. And what was,
indeed, our newly discovered “cross correlation”
variable (D) turns out to be a well known parameter of
curved movement: affine velocity.Â

Â

                MT: I think this is, to put it
mildly, a little different from what Rick said above
that I showed.

              RM: I really tried to find some relevance of your
mathematical analysis to the research we described in
our power law paper. But I’m not sure there is any
relevance because you don’t seem to understand – or
want to understand – what we did. This is evidenced
by what you say at the beginning of your mathematical
critique of our work:Â “Accordingly, they assert that
measured values of the power law that depart from 1/3
are in error because they omit consideration of D”. In
fact, we never “asserted” this. What we demonstrated
is that measured values of the power law coefficient
will depart from 1/3 (for the relationship between R
and V and 2/3 for the relationship between C and A) to
the extent that the variable D, which power law
researchers always omit from the regression analysis,
covaries with the curvature variable (R or C) that is
included as the predictor variable in the analysis.Â

                MT: Try again, Rick. I keep hoping to be able to
learn something from one of your postings, but I
haven’t won this lottery jackpot yet.

              RM: Sure, I'll try again. But you might have better
luck if you would explain, as clearly as possible, how
your mathematical analysis relates to our regression
analysis of actual data from curved movements.

Â

                MT: If I have made a
mathematical error in my other comments on Marken
and Shaffer, I really would like to know. But you
please comment on what I wrote, rather than on
something you invented, as you did in this case.

              RM: As I said before, I don't think you have made
any mathematical errors. I just don’t see the
relevance of your mathematical analysis to what we
actually did with our analysis of actual curved
movement data. Did we use the wrong formulas to
calculate instantaneous velocity and curvature? Did we
do the regression incorrectly? Did we use the wrong
variables in the regressions?Â

Best

Rick

Â

                    Martin
so thatÂ V
= R^1/3D^1/3 =Â R^1/3
V*(1/(R^1/3))
which, of course,Â reduces
to V=V.Â

                              RM:
But as I’ve said, that’s true of any
equation. The fact thatÂ V
= R^1/3D^1/3 can be reduced toÂ V =
V doesn’t negate the value of
knowing thatÂ V
= R^1/3D^1/3. This equation
analyzes V into its components
just as simple one way analysis of
variance (ANOVA) analyzes the
total variance in scores in an
experimentÂ (MS.total) into two
components, the variance in scores
across (MS.between) and within
(MS.within) conditions, so that
MS.total = MS.between + MS.within.
This is the basic equation of
ANOVA.Â

                                  RM:
Of course, it’s possible to show
that MS.total
= MS.between + MS.within isÂ a
“tautology”:Â MS.total = MS.total. We can
do this by noting that MS.within =
MS.total - MS.between so thatÂ MS.total
= MS.between + MS.total - MS.between
which, you’ll note, reduces toÂ MS.total
= MS.total.

                            RM:Â  But by analyzingÂ MS.total
intoÂ MS.between and MS.within we can
learn some interesting things about the
data by computing the two variance
components of MS.total and forming the
ratio MS.between/MS.within, a ratio
known as F (for Sir Ronald Fisher, who
invented this analysis method and, as
far as I know, never caught flack from
anyone about the basic equation of ANOVA
being a tautology). Knowing the
probability of getting different F
ratios in experiments where the
independent variable has no effect (the
null hypothesis), it is possible to use
the F ratio observed in an experiment to
decide whether one can reject the null
hypothesis with a sufficiently small
probability of being wrong.Â

                                                  RM:
Just as it has
proved useful to
analyze the total
variance in
experiments ( MS.total) Â into
variance component
( MS.between,
MS.within and
sometimes
MS.interaction
and MS subjects)
it proved useful
to us to analyze
the variance in
the velocity, V,
of a curved
movement into
components, R
and D. This
analysis
produced the
equation V =
R^1/3*D^1/3. R
and D are
measures of two
different
components of
the temporal
variation in
curved movement
just as
MS.between and
MS.within are
measures of two
different
components of
the variation in
the scores
observed in an
experiment; R is
the variation in
curvature and D
is the variation
in affine
velocity.Â

                                                    RM:
Our equation
says that the
variation in V
for a curved
movement will be
exactly equal to
R^1/3D^1/3.
Linearizing this
equation by
taking the log
of both sides we
get log (V) =
1/3log (R)
+1/3*log (D) .
This equation
shows that if
one did a linear
regression using
the variables
log(R) and
log(D) as
predictors and
the variable
log(V) as the
criterion, the
coefficients of
the two
predictor
variables would
be exactly 1/3
with an
intercept of 0.
More
importantly,
this equation
shows that if
the variable log
(D) isomitted
fromÂ the
regression, the
coefficient of
log(R) will not
necessarily be
found to be
exactly 1/3 and
the intercept
will not
necessarily be
found to be
exactly 0. This
is where Omitted
Variable Bias
(OVB) analysis
comes in. This
analysis makes
if possible to
predict exactly
what a
regression
analysis will
find the
coefficient of
log(R) to be if
log(D) is
omitted from the
regression.

                                                    RM:
This finding is
important
because the
“power law” of
movement is
determined by
doing a
regression of
log (R) on log
(V) using the
regression
equation log (V)
= k + b*log(R),
omitting the
variable log(D).
The term “power
law” refers to
the fact that
the results of
this regression
analysis
consistently
finds that the
power
coefficient b is
close to 1/3.
Our analysis
shows that this
is a statistical
artifact that
results from
having left the
variable log(D)
out of the
regression
analysis. OVB
analyiss shows
that the amount
by which the b
coefficient is
found to deviate
from 1/3 depends
on the degree of
covariation
between the
variable
included in the
regression (log
(R)) and the
variable omitted
from the
regression
(log(D)). Since
both log (R) and
log (D) are
measured from
data (temporal
variations in
the x,y position
of the curved
movement) the
covariation
between these
variables is
easily
calculated and
the predicted
deviation of the
power
coefficient, b,
from 1/3 can be
exactly
predicted.Â

                                                    RM:
The covariation
between log (R)
and log (D)
depends on the
nature of the
curved movement
trajectory
itself and has
nothing to do
with how that
movement was
generated. It is
in this sense
that the
observed power
law is a
“behavioral
illusion”, the
illusion being
that the
relatively
consistent
observation of
an approximately
1/3 power
relationship
between the
curvature (R)
and velocity (V)
of curved
movements seems
to reveal
something
important about
how these
movements are
produced, when
it doesn’t.Â

                                                    RM:
So the fact that
the equation V =
R^1/3*D^1/3 can
be reduced to V
= V does not
negate the value
of analyzing V
into its
components any
more than the
fact that the
equationÂ MS.total
= MS.between +
MS.within can
be reduced toÂ MS.total
= MS.total
negates the
value of
analyzing
MS.total into
its
components.Â

                                                      RM:
There are many
other
incorrect
conclusions in
your rebuttal
to our paper,
Martin. But I
think this is
enough for now
since your
“tautology”
claim (based
on our alleged
mathematical
mistake)
seemed to be
central to
your
argument.Â

BestÂ

Rick

                              You can do this without referring
either to your rebuttal or to the
eight falsehoods that I asked you not
to try to justify at this point. My
question is not about them, but
specifically about what in my
mathematics you have shown to be
bogus. Your previous response did not
address this question.
                              Martin

–
Richard
S. MarkenÂ

                                                    "Perfection
is achieved not
when you have
nothing more to
add, but when
you
have
nothing left to
take away.â€?
Â
Â Â Â Â Â Â Â Â
Â Â Â Â Â Â Â
–Antoine de
Saint-Exupery

–
Richard S. MarkenÂ

                                      "Perfection
is achieved not when you have
nothing more to add, but when
you
have
nothing left to take away.â€?
Â
Â Â Â Â Â Â Â Â Â Â Â Â Â Â Â
–Antoine de Saint-Exupery

MartinT · July 25, 2018, 1:53pm

[Martin Taylor 2018.07.25.09.20]

Why do you keep repeating this lie? If you have read ANYTHING I have written on the subject in the last

many months, or even if you had simply read my published critique,
you absolutely know that it is not true, So why say it again and
again?
Let’s make the actual criticism clear to all the CSGnet readers.

···

On 2018/07/24 10:54 PM, Richard Marken
( via csgnet Mailing List) wrote:

rsmarken@gmail.com

[Rick Marken 2018-07-24_19:54:03]

[Martin Taylor 2018.07.24.13.05]

              MT:
Rick, Do you intend to respond to my last message of
four days ago? Are you going to apologise and
acknowledge that my maths is not “bogus” or are you
going to explain to me exactly how it is bogus?

RM: I thought I answered this but I’ll try again.

          RM:  I said your math was "bogus" because it is
irrelevant to our analysis of the power law. Our analysis
is based on the computational formulas for the curvature
and velocity variables that go into the regression
analysis used to determine whether or not a movement
trajectory is fit by the “power law”. Your math was based
on the fact that the derivatives in the physics equation
describing the velocity of a curved trajectory are taken
with respect to time while derivatives
in the physics equation describing the curvature of a
curved trajectory are taken with respect to space.

³

MartinT · July 29, 2018, 3:28pm

[Martin Taylor 2018.07.29,10.41]

Why do you say that it isn't?

Exactly!!!!!!!

Since you have carefully read, and mathematically understand my

published equations, why on earth do you say that your equation (3)
is not of the form

![CancelFactor_V.jpg|196x58](upload://kAj04l3NItiJbNfqcJH5sVtl7Kv.jpeg)Â Â Â Â Â 

It's simple straightforward first-year calculus, no tricks involved

at all. Indeed, f1(.) turns out to be the identity function, which
makes it even easier.

f2(s, x, y) is simply (dx/ds)(d<sup>2</sup>y/ds<sup>2</sup>)-(dy/ds)(d<sup>2</sup>x/ds<sup>2</sup>    ).

So, because V<sup>3</sup> cancels out, leaving R a simple function

of spatial variables, as normal physics says it must be, any and all
values of V will give the correct result, and nothing whatever about
R can be used to say anything at all about the value of V.
Experimenters have a useable velocity profile at hand from which to
compute R, so they use it. That doesn’t allow them, or you, to
back-compute the “true” velocity from their computed value of R.
Since the rest of your paper (and the rest of the message to which I
am replying) is based on doing just that, none of what you did is
mathematically valid.

I have to admit that my first thought about the nature of your error

was your use of the time derivative in computing R, but Alex
corrected me very quickly. That there was a serious error was
immediately obvious from purely physics considerations, and I had
jumped to conclusions too quickly. But I corrected that over a year
ago, and have never since suggested that you were wrong in using
time derivatives.

Martin

···

[Rick Marken 2018-07-25_10:09:41]

[Martin Taylor 2018.07.25.09.20]

MT:Â Why do you keep repeating this lie?Â

Â RM: Because it’s not a lie.

            MT: If you have read ANYTHING I have written on the
subject in the last many months, or even if you had
simply read my published critique, you absolutely know
that it is not true, So why say it again and again?

          RM: I have read your stuff very carefully and what I

say above is precisely true!

Â

            MT: Let's make the
actual criticism clear to all the CSGnet readers.

RM: Yes, let’s!

Â

            MT: If you have an equation







            MT: z cancels out of the equation. It is therefore
impossible to say anything about a relationship between
x and z.

Â RM: You betcha.Â

            MT: This is the case
for the equations used by Marken and Shaffer:

          RM: This is only one equation and it is not one of the

ones used by Marken and Shaffer.

                            MT:
Rick, Do you intend to respond to my
last message of four days ago? Are you
going to apologise and acknowledge that
my maths is not “bogus” or are you going
to explain to me exactly how it is
bogus?

                        RM: I thought I answered this but I'll

try again.

                        RM:Â  I said your math was "bogus" because

it is irrelevant to our analysis of the
power law. Our analysis is based on the
computational formulas for the curvature and
velocity variables that go into the
regression analysis used to determine
whether or not a movement trajectory is fit
by the “power law”. Your math was based on
the fact that the derivatives in the physics
equation describing the velocity of a curved
trajectory are taken with respect to time
while derivatives
in the physics equation describing the
curvature of a curved trajectory are taken
with respect to space.Â

Â

MT: Since V³
cancels out of the equation, R can be written as a
function only of s, x, and y, and it is therefore
impossible to say anything about a relationship between
V and R.

RM: Yes, that is true for that equation.

Â

            MT: In my published
comment equations 1, 2, 3, and 7, which Marken has
accepted as correct, all make this same point.Â

          RM: Right. It is a point that is completely irrelevant

to our analysis. Our analysis is based on the
computational formulas that are used to compute the values
of the instantaneous velocity and curvature variables that
go into the regression analysis that is used to determine
whether the data are fit by a power law. The computational
formulas are as follows:

        RM: These are the formulas used to compute the velocity

(V) and curvature (R) at each instant during a curved
movement. The derivatives are all computed with respect to
time. Actually, what is computed is an approximation to the
time derivative.

        For example, the time derivative of movement in the X

direction in these equations, X.dot, is computed as
[X(t)-X(t-tau)]/tau where X(t) and (X(t-tau) are the
recorded positions of the movement in the X dimension at
time t and time t-tau, tau being the sampling interval.Â

RM: From equations (2) and (3) we can see that:

and, rearranging terms we get:

RM:

This equation describes the mathematical relationship that
exists between the computed values of V and R.
That relationship is also turns out to be a function of
the computed value of another variable, D, which we called
the cross product variable but, as we learned later from
the power law literature itself, is measure of affine
velocity.Â

RM:

Note that there is nothing in our maths that looks
anything like your equation above.Â

RM:

But, again, I know that you are not going to give up on
this. So how about doing something more productive, like
telling me your explanation of the power law.Â As I said
before, I think you will have a lot more luck convincing
me that my explanation of the power law (as a
statistical artifact) is wrong if you can tell me what
the explanation is that you think is right.Â

Best

Rick

            Children are (or were) taught early
in their math education about an easily made mistake
that allows you to prove 1=2. The proof involves using a
fraction x/y, which is legitimate except when x = y = 0
(or infinity), and if the proof is written
appropriately, it may not be obvious that you have used
0/0. Marken and Shaffer’s error, though they did not use
0/0 literally, is of this same “concealed” class. It has
nothing whatever to do with using time derivatives.

Â

–
Richard S. MarkenÂ

                                  "Perfection

is achieved not when you have
nothing more to add, but when you
have
nothing left to take away.â€?
Â Â Â
Â Â Â Â Â Â Â Â Â Â Â Â Â
–Antoine de Saint-Exupery

MartinT · July 30, 2018, 5:11am

[Martin Taylor 2018.07.30.01.08]

You are going the wrong way around, because any expression for R

must be expressed in spatial variables alone. The point you want is
to demonstrate that this is the case for the expression that starts
with velocities and accelerations, which are expressions in space
and time. The usual way to get there is to start from the expression
in s, x, and y as did Viviani and Stucchi (whom Gribble and Ostry
used as their source – Marken and Shaffer used Gribble and Ostry to
justify their equation) and work from there to the equation labelled
(3). But I’ll work it backwards for you.
in general, dx/dt = (dx/ds)(ds/dt) and dx/dt
= (ds/dt)(ds/dt) and ds/dt = V
Numerator of (3):
(dx/dt)+(dy/dt) = (dx/ds)*(ds/dt)

(dy/ds)(ds/dt)
Â Â Â Â Â = (ds/dt)((dx/ds)+(dy/ds))
Â Â Â Â Â = V* f1(s, x, y)
Â Â Â Â Â But since dx+dy = ds, f1
turns out to be the identity function, the multiplier 1. The 3/2
power in the numerator doesn’t affect the identity function but
turns V into V.
Denominator of (3):
(dx/dt)(dy/dt)-(dx/dt)(dy/dt)
= (ds/dt)((dx/ds)(dy/ds) - (ds/dt)(dy/ds))
Â Â Â Â Â Â = Vf2(s, x, y) where f2 is (dx/ds)*(dy/ds)

(ds/dt)(dy/ds)
Cancelling V out of the fundamental spatial form of the
numerator and denominator leaves
R = 1/((dx/ds)(dy/ds) - (ds/dt)(dy/ds))
It’s worth taking a moment to see at least qualitatively why this
expression (dx/ds)(dy/ds) - (ds/dt)*(dy/ds)
represents curvature (defined as 1/R).
If x changes by âˆ‚x, the acceleration of y describes how fast the
curve increases its rate of change of height as âˆ‚x increases, and
conversely for any increase in the rate of change of change of x as
âˆ‚y increases. Both signify bends, and together they describe bends
in orthogonal directions. It takes a bit more than that to show that
the result does actually describe the radius of curvature and that
it is independent of the initial orientation with which you start,
but I think those two considerations put together should help you to
picture how it could work (as in fact it does). You don’t need any
time or velocity expression to compute curvature (or radius of
curvature. You just need these two products that deal with how fast
x and y change as you look further and further along the curve.
Martin

···

On 2018/07/29 7:38 PM, PHILIP JERAIR
YERANOSIAN ( via csgnet Mailing List) wrote:

pyeranos@ucla.edu

In what way do you get from these equations:

to this equation:

      I see that R = V^3/what looks like some chain rule

expression.

Can you please explain how you see V^3 in the denominator?

²²²²²

²²²²²²
²²²
²
²²²²³

²²²²³²²²²
³²²²²

³

²²²²

      On Sun, Jul 29, 2018 at 3:25 PM,
Richard Marken csgnet@lists.illinois.edu
wrote:
              [Rick Marken
2018-07-29_15:20:10]

[Martin Taylor 2018.07.29,10.41]

                              RM:... Our analysis is based on the
computational formulas that are used
to compute the values of the
instantaneous velocity and curvature
variables that go into the regression
analysis that is used to determine
whether the data are fit by a power
law. The computational formulas are as
follows:

MT: Exactly!!!

                Â RM: Excellent. So it's settled. V = R^1/3*V^1/3.
Or, in linear form log (V) = 1/3* log(R) + 1/3*log
(D).Â

                  MT: Since you
have carefully read, and mathematically understand
my published equations, why on earth do you say
that your equation (3) is not of the form
                  Â Â Â Â Â 

RM: Because it’s not. It’s of the form:

Â

                RM: Which is the form used to calculate R from
the data. Your equation is not the equation used to
calculate R in power law research.Â

                    f2(s, x, y) is simply (dx/ds)(d<sup>2</sup>y/ds<sup>2</sup>)-(dy/ds)(d<sup>2</sup>x/ds<sup>2</sup>                      ).

RM: So how do you calculate ds from the data?Â

                  MT: So,
because V³ cancels out, leaving R a
simple function of spatial variables, as normal
physics says it must be,

                RM: What physics? I think there is only
mathematics involved here, the mathematics of curved
movement trajectories. In the actual production of
curved movements, the physics involved is that which
relates muscle and external forces to the resulting
curved movement. The curved movement that results is
characterized by it’s velocity and curvature at each
point in the movement. There is no physical
relationship between the curvature and velocity of
the movement.Â

Â

                  MT:
Experimenters have a useable velocity profile at
hand from which to compute R, so they use it.

                RM: Experimenters don't use a velocity profile to
compute R. The velocity profile of the movement is
completely invisible to theÂ regression
analysis used to determine the power law. Once you
have computed the paired values of V and R (using
equations 2 and 3) to be used in the regression
they can be entered into the analysis in any
order; in a random order if you like. This is
further proof that the relationship between V and
R has nothing to do with physics.

Â

                  MT: I have to

admit that …Â

                RM: All you have to admit is that you have no
idea what is involved in determining the power law.
And you have no explanation of how organisms produce
curved movement. You just don’t want to believe that
PCT shows that a whole line of research is based on
a mistake. I understand your concern but I just
haven’t got time to care.Â

Best

RickÂ

–
Richard S.
MarkenÂ

                                          "Perfection
is achieved not when you
have nothing more to add,
but when you
have
nothing left to take
away.â€?
Â
Â Â Â Â Â Â Â Â Â Â Â Â Â
Â Â --Antoine de
Saint-Exupery

MartinT · August 4, 2018, 4:03pm

[Martin Taylor 2018.08.04.11.45]

Thanks, Adam, for this message.
For the time being I have personally given up on trying to
explain the issues to Rick, and on reading his messages on the
topic, at least until I think of a new way to address the actual
problem. I hope anyone on CSGnet interested in the
curvature-velocity issue has had enough time to see through the
anti-scientific nonsense he keeps repeating on this issue – not
to mention the self-contradictions and the lies about what is
being criticised, like the one near the end of your quote.

  As for "PCT says", when Rick says it, it is accurate, since as he

has told us more than once, “PCT, c’est moi”.

  Meanwhile, the rest of us can continue to work on the science

involved with the control of perception, and not worry about
whether the science is in accord with what “PCT (Rick) says”.

Martin

···

adam.matic@gmail.com

MartinT · August 13, 2018, 3:52pm

[Martin Taylor 2018.08.13.09.56]

The only effect on the environment available to muscles is the

application of force to accelerate masses or to maintain velocity in
the presence of friction and viscosity. The corresponding observable
when friction is negligible is acceleration. Changing forces are due to changing muscle tension, a controllable
perception. If perception of position is controlled, that control
can be effected only by sending changing reference values to the
muscle tension perceptual control system, however that might be
constructed. After a step change in the position reference value,
even if acceleration were to start at a high value, the initial
velocity would still grow from zero. At the end of the movement some
countering force must be produced to create the opposed acceleration
that brings the velocity to zero when the position perception
matches its new reference value. The velocity profile after a step
change in reference will be a rise followed by a fall, with a
possible intermediary steady state of travel.
Muscles have mass, so even after the appropriate nerves fire, it
takes time for them to accelerate and decelerate into their new
tension that provides the force to accelerate the environmental
mass. Not only will the force acceleration not change abruptly,
neither will their jerk. Jerk, too, will increase and decrease over
the course of the movement to the new position reference value. In
this environment with negligible friction, even the fourth
derivative of position will not change instantly. After a step
change in the position reference, the velocity profile is not a set
of straight lines up-(possible flat)-down, but curves smoothly, not
as a square-law parabola but as a third-power curve, into the three
changes of slope.
In an environment in which friction dominates mass, the argument is
the same, except that it is the velocity of the moving environmental
object, rather than its acceleration, that is influenced by changes
in muscle tension. Acceleration effect due to mass would be
effectively instantaneous, but changes in velocity (observed
acceleration) would depend on the accelerations of the muscle masses
exactly as in the negligible friction case. Martin

···

On 2018/08/13 5:50 AM, Adam Matic
( via csgnet Mailing List) wrote:

adam.matic@gmail.com

[From Adam Matic]

…
BP: This is purely a consequence of the mathematical
relationships of control and the passive dynamical
properties of the arm; nothing is acting to make sure
that the trajectory follows any particular path. ** The
trajectory is a side-effect** , not a planned
movement. Evidence of trajectory planning would appear
only if the actual trajectory departed from the one that
can be explained as a step-change in the reference
signal of a control system from one fixed value to
another.

          AM: He also said that velocity profiles are a side
effect, which is equivalent to saying that the trajectory
is a side effect. I have no idea what you mean by “a
trajectory profile”. He did not say that there is control
of instantaneous position of movement - there is control
of position, but only with step changes in the reference.

MartinT · August 15, 2018, 3:14pm

[Martin Taylor 2018.08.15.10.41]

…

        I'm not going to comment on your affine velocity musings,
I don’t understand what affine velocity is, and it looks to
me you don’t know how to define it so it makes sense. The
experiment looks simple enough that you can do it yourself.

Going back a few months…

[Martin Taylor 2018.03.17.13,45]
(Replying to [Rick Marken 2018-03-17_09:45:00])
  As you said, "affine velocity" is the D in your paper. You cite
Pollack and Shapiro, but not my simpler way of doing the maths
they do to show that D is easily decomposable into V time a pure
function of geometric variables, and is therefore of no value in
an expression that purports to explain or describe the value of V.
It is not clear why you cite Pollack and Shapiro approvingly, but
fail to approve my criticism, instead refusing to mention it in
your rebuttal, instead substituting an entirely different
criticism that you pulled out of thin air. If you believe Pollack
and Shapiro, you have to believe me, too. You can’t have one
without the other.

To which Rick made one of standard-type replies that was apparently

intended to contradict the foregoing, but instead simply affirmed
that he was correct, while carefully not addressing my point. My
point was that Pollack and Shapiro produced the same analytical
result as I had, and that therefore Rick could not logically extol
the virtue of their analysis that he did not understand while
discarding my analysis (which he apparently has also never
understood). Either analysis is in itself a sufficient refutation of
the Marken and Shaffer paper without the need for any of the other
criticisms that he has never answered, despite claiming to have
answered them when he answered criticisms he invented out of thin
air.

For Rick to cite Pollack and Shapiro or "affine velocity" in support

of his nonsense is chutzpah of a level seldom approached.

------affine velocity------

In this context, intuitively you can explain "affine velocity" (not

a technical term in itself) quite easily. Suppose you have a circle
lying on the ground some distance away from you. From your off-axis
viewpoint it projects to your eye as an ellipse (an affine
transformation), and would do so no matter how far the circle is
slanted away from you. If a marker point moves at constant speed
around the circle, its projection moves more slowly around the tips
of the projected ellipse (toward and away from you on the circle)
than it does when it moves across your line of sight along the wide
sides of the projected ellipse. If I remember correctly, Pollack and
Shapiro showed that the projected (“affine”) transform of a velocity
that is uniform around the original circle conforms to the 1/3 power
law on the projected (“affine”) circle that is the ellipse.

Martin

MartinT · August 16, 2018, 5:07am

[Martin Taylor 2018.08.16.00.42]

[From Erling Jorgensen (2018.08.15 2345 EDT)]

Erling Jorgensen (2018.08.15 1830 EDT)

Rick Marken 2018-08-15_18:54:36

EJ: I am suggesting a different PCT explanation than the statistical-

artifact/illusion one that Rick is proposing.

RM: The fact that the power law is an illusion can be determined without any

knowledge of statistics.

EJ: I like how you insert the word “fact�? about what is indeed a proposal
still being contested.

Actually, the various power laws are experimental observations. They are not illusions. I don't think that statement is a proposal, it's just that the power law is what many people have reported they observed, so I suppose that the power law is an observation, not an illusion, is as close to a "fact" as you are likely to get.

On the other hand, since at least a fly larva is unlikely to be perceiving the value of the power that relates the velocity of its movements to the radius of curvature of its movements, the observed power law (at least in that case) is almost certainly a side-effect of control. Personally, I should be very surprised if it were found that a human or any other animal was perceiving the power and correcting it during the tracing of either an ellipse or a free-form scribble.

It's nice that Rick has come around to the idea that finding what variable(s) are controlled when fly larva or other organisms create curved trajectories at speed. When he first proposed his mathematical nonsense I argued that the first thing to do was to find the controlled variable(s), but he seemed to reject the idea that this was at all necessary, since he had already solved the problem. Even after nearly two years I still say find the controlled variable(s), find the mechanism (muscles, eyes) and the physical constraints such as mass and viscosity, and with luck you will find why controlling those variables have those side-effects.

At some point in the output side of the chain, there must be a changing reference position, which the moving object will attempt to follow. But that's not interesting. The issue is why that reference value changes the way it does, and how the actual control system deviates from the reference. In [Martin Taylor 2018.08.13.09.56] I argued that with a step change of position reference, the actual trace in a friction-free environment would have a changing fourth derivative (at least), and in a high friction (high viscosity) environment it would have a changing third derivative (jerk) at least. These do constrain the shape of the trajectory. Whether they are relevant to the power-law side effect of moving over a curved path is anyone's guess.

Martin