I find the progress of e coli reorganization is similar to a math method called radom walk which have been fully developed in the last century. I also notice that rick and bill published a paper in 1989 using the word “biased random walk” in the descussion of e coli reorganization. I believe there’s something worth to learn in order to push the study of reorganization.
This is a quote from a paper named “ROBUST VARIANCE REDUCTION FOR RANDOM WALK METHODS”:
Random walk methods are effective for solving linear partial differential equations in
many dimensions, especially those involving complex geometries.
It’s just what e coli is doing.
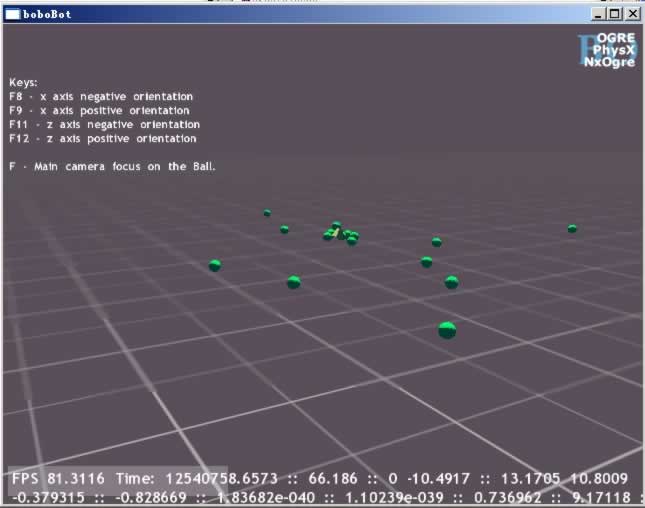
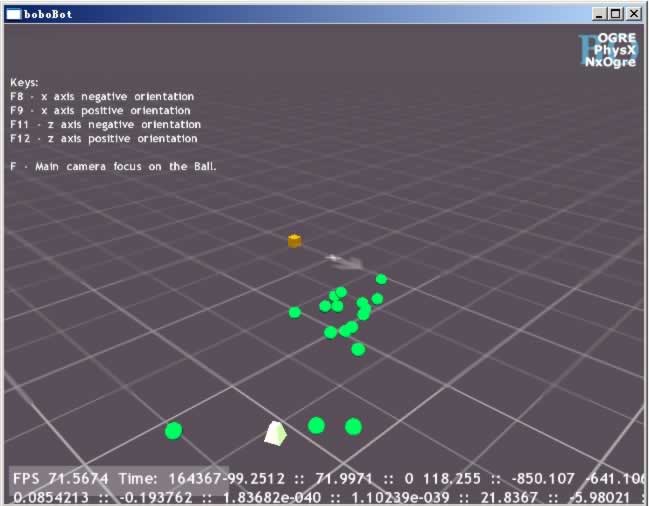
I built a new programme these days with my “Physics lab”(The platform I showed my cricket). Some green balls evolve to chase a white convex. These green balls don’t have any ability at first, then e coli reorganization method(I don’t know whether the specific method I used is the same with Powers’, I
programmed it in my understanding) finds appropriate weights for each ball. Since the evolution is totally random, every ball finds its own way to get close to the target convex. Some go straight and some go in a curve(the number of balls going on both sides are almost same). Some are faster than trains while some are slower than snails.
Most of them get to the final goal - a sufficient control system. When I move the target convex to any place, they could chase it. In the progress, there’re some interesting phenomenas. Some balls gave up because the target was moving faster than them. Some balls ran far away at the begining, but they suddenly accelerated in the target’s direction and stoped beside it.
The whole looks like a shoal chasing crumbs. This also remind me that it’s simliar to photons. A single one doesn’t means anything while lots of objects show some common characteristics. Files attached are 2 screenshots, the programme is too big to send in a
mailing list, I’ll put it on web some day and you can try it on your own machine.