[From Rick Marken (2014.12.03.1845)]
Fred Nickols (2014.12.02.1330 EST)–
RM: I believe I have finally “solved” the problem of control of behavior. I am busy right now and won’t have time to give a detailed explanation until later but I just wanted to give you what I think should be a tantalizing preview of my solution.
FN: Great! Can’t wait!
RM: OK, here we go.
RM: Control of behavior is an illusion in the same sense that stimulus-response behavior is an illusion. The latter is the so-called “behavioral illusion” and it refers to the fact that abrupt disturbances to a controlled variable appear to be a stimulus that causes the resulting compensating response via the organism (see the “A Bucket of Beans” paper in LCS II). A linear analysis of of a control system shows that the causal path between stimulus (disturbance, d) and response (output, q.o) runs through the environment, being the inverse of the environmental feedback connection, k.e, between system output, q.o, and controlled variable.
RM: So the stimulus-response law for a control system is q.o = r - (1/k.e)*d, where r is the system’s reference. The apparent causal relationship between stimulus (d) and response (q.o) is determined by 1/k.e, a property of the environment, not of the system itself. (While r is a property of the system, it is independent of the relationship between q.o and d and therefore doesn’t affect the apparent causal relationship between . these variables).
RM: The behavioral illusion turns out to be the the basis of the illusion of control of behavior. My analysis is illustrated in the figure below. But before I get to explaining that let me briefly discuss the meaning of the term “illusion”. In perceptual psychology an illusion is a perception that we know, for various reasons, does not correspond to reality. For example, in the Ames room illusion (www.youtube.com/watch?v=hCV2Ba5wrcs) we see people change size as they walk across the room. We consider this an illusion because we know that people don’t change size as they walk from one place to another.
RM: This means that some things that are called “illusions”, such as Ellen Langer’s “illusion of control”, are not illusions at all but, rather, misconceptions. For example, Langer would call a gambler’s belief in his ability to control the spots that come up when the dice are thrown an example of the illusion of control. But the gambler doesn’t really see himself controlling the dice; he is just mistaking coincidence for control.
RM: The control we see in control of behavior is, however, a true illusion, as is the behavioral illusion. In both cases we see something happening (just as we see a person shrink and grow in the Ames room) but we know, for other reasons, that what we see is not what is actually going on. In the case of the Ames room we know what we are seeing can’t really be happening based on our understanding of the physical model of reality. And in the case of the behavioral illusion and the illusion of control of behavior we know (or should know) that what we are seeing can’t really be happening ased on our understanding of control theory.
RM: So my mistake in arguing that “control of behavior” actually happens was to take appearance (of control of behavior) for reality. I was wrong for the right reason. I was right that control of behavior is an observable (and measurable) fact, just as the change in the size of a person walking across the Ames room is an observable (and measurable) fact. But control of behavior is not actually happening any more than the response that results from a sudden disturbance to a controlled variable is actually a caused response (or that the person walking back and forth across the Ames room is actually shrinking and growing).
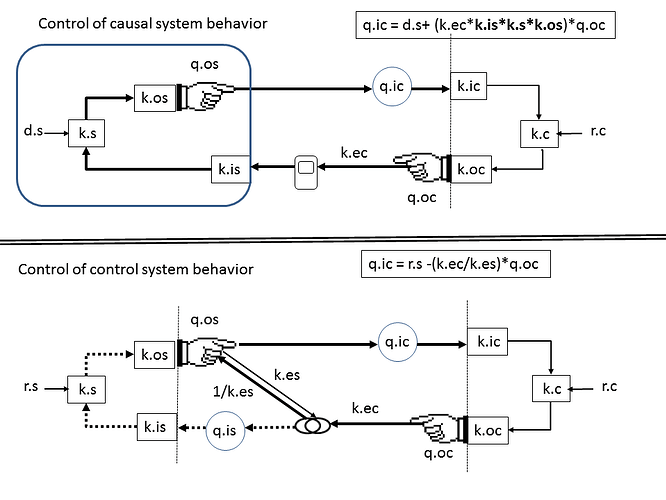
RM: The diagrams below explains the difference between “real” and illusory control of behavior. The diagram at the top shows the “real” control of the behavior of a cursor on a computer screen and the one at the bottom shows the illusory control of the behavior of a control system. In both diagrams the controller (system c) is on the right and the system whose behavior is being controlled (system s) is on the left.
RM: In the top diagram system s – the system whose behavior is being controlled – is a causal system because inputs from mouse movements, which are the outputs of system c, q.oc, are the cause of the system s output, the behavior that is controlled; the output of system s that is controlled is the pointing finger cursor displayed on the computer screen, q.os.
RM: I’ve drawn the causal system to look a bit like a control system but it’s not. The variable d.s looks like a reference signal but it’s actually the computer generated disturbance that is added to the controller’s input to produce the displayed output.
RM: The fact that system s is a causal system is shown by the equation at the top right of the top diagram. It’s the equation that describes the causal connection between the inputs to system s from the system c, q.oc, and the outputs o system s, q.os, which are the input, q.ic controlled by system c. The equation is the feedback function that relates system c’s outputs, q.oc, to its inputs, q.ic.
RM: The bold arrows leading from system c’s output, q.oc, its controlled input, q.ic, is the causal feedback path from the controller’s output to the controller’s input. What is most important about this feedback path is that it goes through system s, the system whose behavior (cursor position, q.os) is being controlled. This means is the controller’s (system c’s) outputs are one cause of the controlled system’s (system s’s) outputs (the other cause of system s’s output is the disturbance, d.s, generated by the computer).
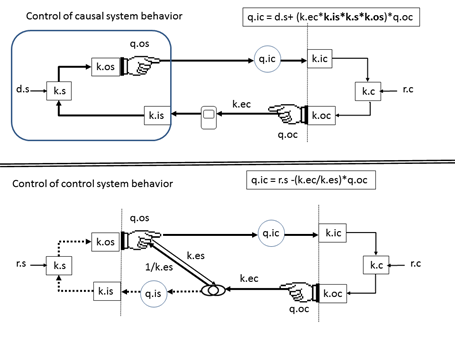
RM: In the bottom diagram, system c – the system whose behavior is being controlled – is now a control system, controlling q.is, which corresponds to the position of the knot in the rubber bands. The outputs of the system c, controller, q.oc, no longer have a direct effect on the inputs system s; rather they are a disturbance to the variable controlled by system s, q.is. This means that, unlike in the top diagram, the feedback path from the controller’s outputs, q.oc, to the controller’s input, q.ic, does not go though the system being controlled. In this case the feedback path for the controller goes through the environment only. This is shown by the equation in the upper right corner of the lower diagram and by the solid arrows in the diagram.
RM: The equation is the feedback function for the controller (system c) and it shows that none of the coefficients that relate the controller’s output to the controller’s input are part of system s, the system whose behavior is being controlled. The coefficients k.ec and k.es are properties of the environment that determine the effect on the knot (the controlled variable, q.is) of the output of the controller (system c) and the system under control(system s), respectively. The solid arrows shows the feedback path graphically; the path never goes through system s, the system whose behavior is being controlled.
RM: The difference between the top and bottom diagrams in the figure above describes the illusion of control of behavior. Both diagrams show a controller, system c, controlling a behavioral variable, q.os, which is the position of the pointing finger. In both cases an observer would see q.os being kept under control. But the control seen when the behavior under control is that of a causal system is not the same as the control seen when the behavior under control is that of a control system.
RM: If one sees the control of the behavior of the control system as being like that seen in the upper figure, then that is the illusion of control. It is an illusion because the control you are seeing is not like the control of the behavior of a causal system. The difference turns on the nature of the feedback function for the controller (system c) in the two cases.
RM: When the control you see is that of the behavior of a causal system the feedback function for the controller runs through that system so that the controller’s output has a direct, causal effect on the variable under control. This is what I would call “real” control. When the control you see is that of the behavior of a control system the feedback function for the controller runs through the environment only so that the controller’s has no direct, causal effect on the variable under control. Indeed, the only influence the controller has on the controlled variable is via the feedback effect, k.es, that the system under control has on the variable it is controlling (q.is). That is, the effect of the controller on the behavior under control (q.os) depends on the fact that the system under control is controlling a variable to which the controller’s outputs (q.oc) are a disturbance. Another way of saying this is that the controller’s ability to control the behavior of a control system depends on the behavioral illusion; the fact that disturbances to a controlled variable will appear to cause outputs.
RM: The apparent causal connection between the controller’s output (q.oc) and input (q.ic) is mediated by 1/k.es, which does not reflect an actual causal link from q.oc to q.ic. That link, from q.oc to q.ic, is the feedback function for the controller (system c) controlling a control system (system s). And since it is not a causal link, the controlling that is going on is illusory in the sense that real controlling (of the kind seen in upper diagram) occurs when the feedback path from system output to input is causal.
RM: Well, that’s a lot of talk but I think teh essence of the thing is contained in that last paragraph. The observed control of behavior of a control system is illusory in teh sense that the controller does not have an actual “hard” causal connection to the variable under control.
RM: Sorry to write so much but I’m thinking of turning this into a little paper for publication and I’m just kind of thinking my way through it. So any “feedback” would be most welcome. But I think I’ve got the basic idea. Control of behavior is really an illusion (in the sense I describe above) and it results from the fact that the feedback connection to the controlled variable is not causal when the controlled variable is a behavior of a control system.
Best regards
Rick
···
–
Richard S. Marken, Ph.D.
Author of Doing Research on Purpose.
Now available from Amazon or Barnes & Noble
In nature there’s no blemish but the mind
None can be called deformed but the unkind.
Shakespeare, Twelfth Night