[Bruce Nevin (2017.07.12.08:53 ET)]
Martin Taylor 2017.07.11.10.35–
Very interesting, Martin.
In the Powers circuit, the reference signal at level n branches into the perceptual input for level n+1 (when the ‘imagination switch’ is closed). Ditto in my “always on” proposal.
In your proposal, the reference signals at level n-1 (= error output from level n) branch into the perceptual input for level n+1, bypassing the perceptual input function at level n where perceptual signals from level n-1 are combined to construct level n perceptual signals.
Error output from level n branches downward to the reference input of a plurality of controllers below. The reference input there receives input from a plurality of error outputs of controllers above.
I have a hunch this may have unforeseen consequences. It may take some time to puzzle out.
···
On Tue, Jul 11, 2017 at 12:06 PM, Martin Taylor mmt-csg@mmtaylor.net wrote:
[Martin Taylor 2017.07.11.10.35]
On 2017/07/9 6:16 PM, Bruce Nevinwrote:
…
The B:CP view of imagination is that a copy of thereference signal (here, the reference for a missing bit of
input) branches across to create a perceptual input signal
returning to the originator(s) of the reference signal. B:CP
depicts this as a switch actively making and breaking a neural
connection. This has seemed implausible to me, so I proposed
that the imagination signal is always present. When there is
actual perceptual input, the copy of the reference signal
augments it; when there is not, then the copy of the reference
signal provides some (weak) input of that perception.
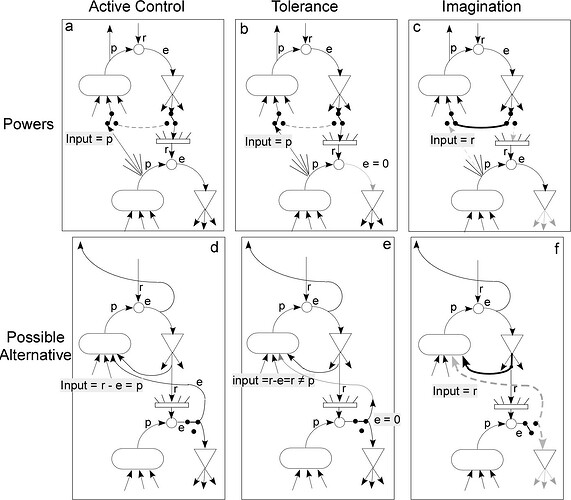
Here's another circuit that produces exactly the same results asdoes the Powers HPCT hierarchy when it is not in imagination mode,
but also does a couple of other things that the Powers circuit does
not do. It has not been tested, but then neither has the Powers
circuit been tested in imagination mode, which is where the two
circuits differ most.The circuit was inspired by one offered in the "Predictive Coding"school of thought (Seth A. K., Friston K. J. (2016), * Active
interoceptive inference and the emotional brain* . Phil. Trans.
R. Soc. B 371: 20160007. http://dx.doi.org/10.1098/rstb.2016.0007 ).
Because I came across the paper in an e-mail exchange with Friston,
I call it the “Friston” connection, though it is not exactly the
same as the one in their paper.Comments: The diagram does not show the distribution of the reference and theerror to other higher-level units (it’s that same as to the one
shown), and the “input = …” notes in the diagram do not take into
account similarly paired inputs from other lower-level units.When the circuit is not in "imagination mode" and the perceptualvalue is outside the tolerance zone, the perceptual values at every
level are exactly as they are in the Powers circuit, with the caveat
that the “error function” is necessarily at least slightly nonlinear
because of the existence of a possible tolerance zone.Some things this circuit does differently from the Powers hierarchy: 1. "the imagination signal is always present", but is, as Powerssuggested, the reference signal for the perception the lower-level
control unit is asked to produce. As in the Powers circuit, the
reference is determined contextually, depending not only on the one
higher-level unit depicted, but on other higher-level units as well.2. In the Powers circuit, it is impossible to perceive a referencevalue, other than by turning the switches and going into
“imagination mode”, and it is impossible to perceive an error value
by any internal means. The alternative circuit allows both to be
used on a par with the incoming sensory-based data as distinct
inputs to higher-level perceptual functions.3. If a control unit has a non-zero tolerance zone (as Bill P. saidthat any well-designed control system should do), and if the
perceptual value is within the tolerance zone, the value that is
reported to the next higher level is the reference for that
perception rather than the actual perception. I don’t know if you,
dear reader, is an amateur musician, but I am, and I find that if I
am playing for myself, I am likely to hear myself playing what I
want to hear, but if I am playing with the knowledge there is a
listener, I hear many more mistakes as my tolerance for them
shrinks. So this effect of the alternative connection seems
plausible to me. It’s not so easy to account for with the Powers
circuit that reports the actual perceptual value to the next higher
Perceptual Function regardless of the width of the tolerance zone.Further comment: This circuit includes only one switch, the one that breaks thelower-level loop and prevents it from controlling its perception,
rather than the two switches in the Powers circuit. We need such a
shut-off in any case, to prevent us from acting out our dreams (or
falling out of a tree) when sleeping.We draw such circuits with single lines that represent paths thatconvey “neural currents”. But a “neural current” is a fiction, a way
of combining the firings on a bundle of neurons into a number that
can be managed in simple calculations. When you think of the lines
as bundles in which the firing rates of the individual fibres
matter, some will be responsive to slightly different inputs from
their incoming synapses than are others in the same bundle. The
effect is to spread the firings over the bundle fibres differently
for clearly perceived and for hazily perceived perceptions that have
similar neural currents. The Alternative Circuit deals with this by
always producing a blend of imagination and sensory data across an
“imagination bundle” (reference value fibres) and a “sensory bundle”
that is represented by the error signal. The clearer the lower-level
perception, the less the higher level unit’s perception uses the
imagination (a.k.a.) reference value by itself because the
concentrated perceptual bundle mates directly with the concentrated
reference bundle rather than meeting it in a defocused way.---------- I'm not proposing this circuit as THE alternative to the Powers HPCTconnection. There are undoubtedly many other possibilities. I just
offer it as a demonstration that we should not get too hooked on the
idea that there’s only one way the hierarchy must work.The Powers connection does lead to questions, as the quote fromBruce illustrates. “* How can we perceive what we want, as we
consciously are able to do?”* is another, as is “* How can we
perceive the difference between where we are and where we want to
be* ?” as we also are consciously able to do. Such questions
should lead us to find circuits that don’t lead to such questions,
or that provide their answers in a simple fashion.If (as Powers claimed) any conscious perception must be representedin the hierarchy as a perceptual value, and if reference and error
values do not exist in the perceptual side of the hierarchy, then if
the Powers hierarchy were literally correct, both of those questions
would have to be answered with the counter-factual: “We can’t do
that.” The alternative I suggest here answers both questions by
placing reference and error separately into the perceptual upgoing
data flow, so that both are potentially available to perceptual
functions at higher levels as separate signals, perceptible and
available to consciousness.Martin