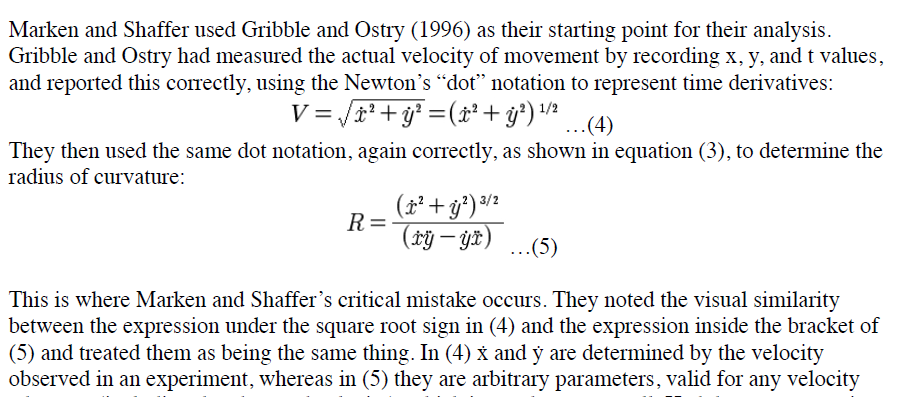
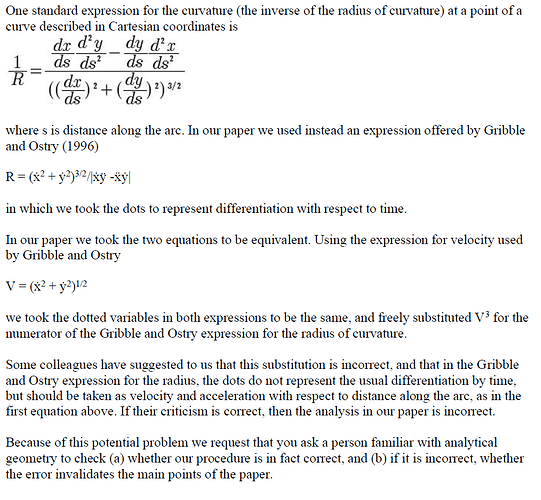
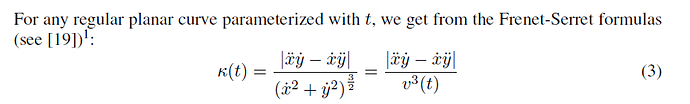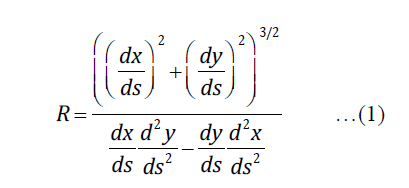This is my attempt to explain why the discussion of the power law of movement is important, to me anyway. It is because the disagreement about how to explain the power law illustrates why PCT has had – and continues to have – so much difficulty being accepted by mainstream scientific psychologists and being understood by those who have accepted it.
The difficulty comes from taking PCT to be a new theory when, in fact, it is a new paradigm. A paradigm is a set of "past scientific achievements that some particular scientific community acknowledges for a time as supplying the foundation for its further practice (T. S. Kuhn (1996) The Structure of Scientific Revolutions, 3rd Ed., Chicago, University of Chicago Press, p. 10). “Further practice” refers to what that scientific community considers the right way to go about their business; it’s “normal science”.
So, a paradigm is the principled basis for the conduct of normal science. It is not a theory but, rather, the context in which different theories are tested. In scientific psychology the current paradigm is what I have called “the causal model” and it is the basis for the conduct of what is considered normal scientific research in psychology; research that is used to test many different theories in many different subdisciplines of psychology.
This causal model paradigm emerged in the early 1900s with the success of Behaviorism. Powers seems to have understood Behaviorism as equivalent to the causal model paradigm because he began his discussion of PCT in Behavior: The Control of Perception by contrasting it to Behaviorism. Unfortunately, the presentation of PCT as a new paradigm was somewhat conflated with his description of PCT as a new theory. But he didn’t conflate theory and paradigm in his Spadework paper (Powers, W.(1978) Quantitative analysis of purposive systems: Some spadework at the foundations of experimental psychology, Psych. Rev., 85, 417-435) when, at the end of that paper he says “Kuhn (1970) uses the term paradigm in the sense I mean when I say that control theory is a new paradigm for understanding life processes—not only individual behavior but the behavior of biochemical and social systems.”
The relevance of all this to the power law is summarized in another quote from Powers’ 1978 paper: “The difficulties faced by a new paradigm, as Kuhn explained so clearly, result not from battles over how to explain particular conceptual puzzles, but from bypassing altogether old puzzles that some people insist for a long time still need solving”. The battles over the PCT explanation of the power law reflect the difficulties faced by this new paradigm. They have been ostensibly over how to explain the power law but, in fact, they have been mainly over whether or not the power law is an “old puzzle” that no longer needed explaining.
The fact that this was the case can be seen in the nature of the reaction to the PCT explanation of the power law. If it were just a reaction to an alternative theoretical explanation of the power law then the battle would have been over which theory best accounts for the data. But the battle was mostly over whether the PCT explanation was scientifically correct, suggesting that the reaction was to a new paradigm; a paradigm that suggested that the power law was a phenomenon that no longer needed explaining.
The reaction to the PCT explanation of the power law has been consistent with what Kuhn ( and Powers) expected it to be; a reaction to a new paradigm as opposed to a new theory. A new paradigm would be seen as not just a wrong theory, but as a wrong way of doing science itself; it would be seen as not normal or not good science. I think this is one of the main reasons why PCT has had such a hard time gaining traction in scientific psychology. To the extent that PCT has gained some apparent traction it has done so by being sold as an alternative explanation of observations that have been made in the context of the current causal model paradigm; the fact that PCT is a completely new paradigm on which to base a new science of psychology has been lost.